Beyond the Event Horizon: An Introduction to Black Holes

Imagine a world in which a beam of light rose into the sky only to fall back to the ground at your feet. Or, picture an infinite continuum of parallel universes, each inhabited by slightly different parallel twins of yourself.Visualize a place in which all the laws of physics, that combine to make our universe the place that it is, vanish into inscrutable infinities. Welcome to the world of one nature's most bizarre phenomena: the black hole.

Prior to 1905, space and time were comfortable absolutes. Over 250 years of practical experience and experimentation had firmly established the supremacy of the physics of Sir Isaac Newton. The picture of the universe painted by Newton was one of amazing clarity and practical value. The motions of projectiles, pendulums, steam engines, and even distant planets could be readily explained using the brilliant 17th century physicist's theories. Certain phenomena, including how light was able to travel in a vacuum and the exact nature of gravity continued to elude satisfactory scientific explanation. While searching for a solution to the failure of the now famous Michelson-Morely experiment (1), Albert Einstein discovered his Special Theory of Relativity. A few years later, Dr. Einstein expanded his theory into an all encompassing grand view of the universe. His General Theory of Relativity was the first to describe the nature of gravity.

The General Theory describes a four dimensional universe in which the three spatial dimensions are coupled with a fourth, time. Any object in the universe with mass is described as causing a warp, or curve into the very structure of space-time itself. Gravity is shown to be a result not of some unseen, mysterious force, yet as a function the curvature of space itself. All matter, from the tiniest sub-atomic particle to the most massive of galaxies, will induce this curvature (2). This idea is frequently explained by describing space as a rubber sheet and a body such as the sun as a bowling ball. If the ball is placed on the rubber sheet, the sheet will bend under the weight of the ball, forming a gravity well (figure 1) . Thus, the orbits of the planets can be seen to result from them "rolling" around the mouth of the sun's gravity well. Of course, it must be realized that this analogy is but a shadow of the true nature of space. In reality, this gravity well is a four dimensional structure.

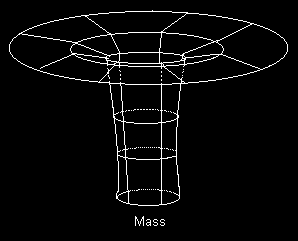
Figure 1: The Rubber Sheet Analogy of Gravity

Shortly after the publication of the General Theory, physicists began to explore this strange new world. The theory is so very complex, and the mathematics so difficult, that even today, scientists have barely scratched the surface of this powerful theory. One of the very earliest solutions to the equations was developed by the German physicist, Karl Schwartzschild, in 1916.

The Schwartzschild Black Hole

Dr. Schwartzschild found that if a mass is compressed into a sufficiently small radius, space-time becomes so severely distorted that even light can't escape from the gravity well. While it may seem improbable that such compression could ever truly occur in nature, it has been found that stars containing, minimally, between two and three times the mass of our sun should collapse in just such a manner. The theory being that once these stars exhaust their supply of fuel, they begin to collapse inward with such tremendous force that even the powerful internuclear forces within the atoms of the star aren't sufficient to prevent it from continuing to fall in on itself until the entire mass of the star is concentrated at a point called a singularity. Within the singularity, matter is infinitely compressed into a region of infinite density. At the singularity, gravity is infinite. Space-time has become infinitely curved (3). At the present time, science has no tools to describe conditions within the singularity. All laws of physics lose meaning in such a region. Schwarzschild's work, however, does shed some light on conditions in the immediate vicinity of this cosmic forbidden zone.

As the star begins to contract, it's mass becomes increasingly concentrated into an ever smaller region of space. The effects of gravity become increasingly pronounced. Soon, the gravity becomes so intense that a beam of light directed out into space will fall back to the ground, following the same parabolic path as an earthly projectile. At a point very near the end of the body's existence as a star, light must be directed perpendicular to the ground in order for it to escape. When the collapsing star passes its Schwartzschild radius, it vanishes from view because its escape velocity has exceeded the speed of light. Since, according to Einstein's work, nothing can travel faster than the speed of light, nothing, not even light itself, can escape from the star. It has become a black hole. The outer edge of the hole is called the event horizon, because no knowledge of events beyond it can ever be passed to the outside world. At a distance of about 1.5 times the radius of the event horizon, lies a region called the photon-sphere, in which the black hole's gravitational pull isn't strong enough to pull light into the event horizon, yet strong enough to prevent it from escaping. Photons of light are forever trapped inside this region, destined to orbit the black hole forever (figure 2) .

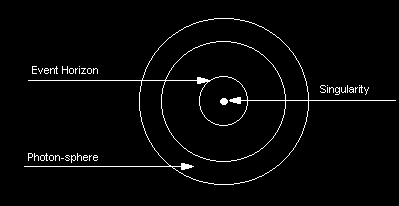
Figure 2: Diagram of Schwarzchild Black Hole

Assuming that we could find a volunteer to journey into a Schwarzchild black hole, what would he experience? Passing through the photon-sphere, he would be flooded with intense light. As our volunteer left the brightness, he would find himself in utter darkness. He would feel his velocity increase to unbelievable levels. Approaching the event horizon, our astronaut would be subjected to tidal forces of astronomical proportions. His feet would seem to weight uncounted trillions of tons more than his head. In a blinding instant, our poor volunteer would be disintegrated into atoms. He would then crash into the singularity, where his mortal remains would be summarily smashed out of existence (4).

Black Holes With Angular Momentum

The Schwarzchild black hole, while fascinating, will probably never be found in nature. Since the holes form from stars, and since all stars are known to rotate, any black hole discovered in nature can be assumed to be spinning. As testimony to the difficulty of finding solutions to Einstein's equations, the equations for a black hole with angular momentum (spinning) weren't discovered until 1963. Roy P. Kerr, an Australian mathematician, accidently found the solutions while working on another problem. The spinning black hole is much like the Schwarzchild black hole, with a few interesting differences.

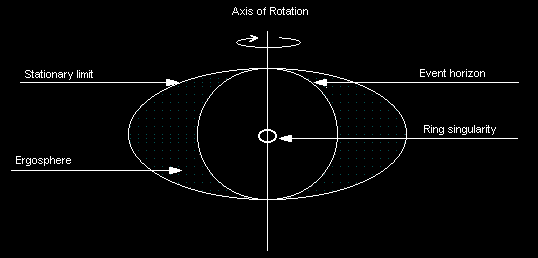
The first thing that a traveler into a Kerr black hole would discover is that he was being dragged around the hole as it rotated. For any spinning black hole, there exists an invisible boundary, known as the stationary limit. Within the stationary limit, nothing can escape being dragged around the black hole - unless it can travel faster than light, currently an impossible condition. At the limit itself, a visitor could avoid being dragged around (and remain stationary), providing that he could travel at the speed of light. The region between the stationary limit and the event horizon is known as the ergosphere. Interestingly, it is theoretically possible for matter to escape from the ergosphere, however, it can't escape being dragged around within it. The ergosphere has an unusual shape (figure 3) . It touches the event horizon at the poles, and stretches out to a distance equal to the radius of the event horizon of a Schwarzchild hole of equal mass (5).

Figure 3: Kerr black hole (6)

Not only does the Kerr black hole exhibit interesting external features, but within the event horizon, it is also different from the non-rotating version. The singularity takes the form of a ring. Physicists have discovered that this singularity is time-like, as opposed to the space-like (7) singularity of the Schwartzchild hole. This means that only objects that entered the event horizon on the plane of the equator would be destroyed in the singularity. Part of Kerr's solution shows that the region bounded by the ring singularity is a region of the most extreme peculiarity. This is a region of negative space-time! While the meaning of this is still being debated, the consensus among many scientists seems to be that this is an area in which gravity is switched into a repulsive, rather than an attractive force. In other words, this is a region in which the presence of a mass in the rubber sheet analogy would cause the sheet to bend upward (figure 4) . Another theory claims that this is a region, within which, objects have a negative radius. Unfortunately, no one can find any physical meaning in such a concept (8).

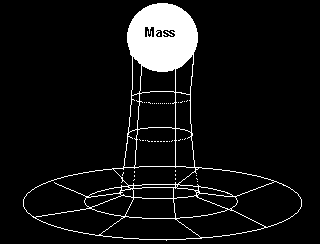
Figure 4: Negative space-time.

The Naked Singularity

One of the most unusual characteristics of a Kerr black hole is the possibility that it could evolve into a naked singularity. Due to the law of conservation of angular momentum, a rotating black hole should rotate ever faster as its radius decreased. Once the object's angular momentum increased beyond it's mass, the event horizon of the hole would be moving in excess of the speed of light. At this point, the event horizon would simply vanish from the universe, exposing the singularity. The absence of the event horizon means that we could travel freely into and out of the singularity. While no one has yet to prove that naked singularities cannot exist, most physicists are strongly inclined to believe that such is the case. Safely within the event horizon, a singularity is effectively shut out of the universe. When it is naked, this region of utter disregard for the known laws of nature is free to interact with the rest of the universe. To illustrate just how disruptive such an object might be, the simple act of going into orbit around a naked singularity would enable one to travel to any point in the past (9).

Parallel Universes

Of all a black hole's bizarre characteristics, none seems stranger than the fact that the solutions to Einstein's equations tell us that these holes in space-time can serve as bridges into other universes. As fans of science fiction are well aware, a parallel universe is a universe entirely separate from our own. Among the many speculations as to the nature of these universes, is the idea that there could be parallel versions of ourselves inhabiting these universes; each living out a slightly different version of our lives. This idea doesn't seem so irrational when viewed in relation to the equally strange world of quantum mechanics (10). It is important to note, however, that the existence of these other universes is, at present, a purely theoretical construction.

Scientists often use a space-time diagram to demonstrate graphically the
strange world of General Relativity (figure 5) .

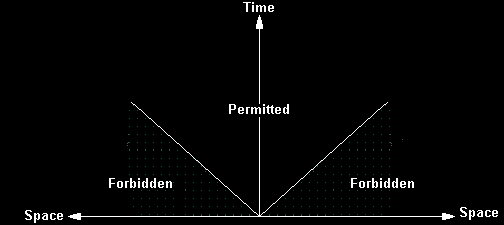
Figure 5: Space-time diagram (11)

The future is located at the top of the diagram. All motion in space must travel within 45º of the vertical time line. Paths that are inclined greater than 45º are space-like, faster than light trips. Accordingly, the zone below the 45º line is shaded gray and marked "forbidden". Professor Roger Penrose of Oxford University has developed a special type of space-time diagram that is very useful for representing the solutions of black hole equations (12). These diagrams quickly show the black hole's connection with parallel universes.

Figure 6 is a Penrose diagram of a simple Schwartzchild black hole. Upon first glance, it appears far more complex than the diagram in figure 5 , yet it really isn't. Just as before, all paths through space must be inclined at an angle less than 45º from the vertical axis. The singularity of the black hole, denoted by the row of shark's teeth at the top, is at a 90º angle, hence it is space-like. The event horizon's one way nature is shown by the sharp bend in it's line. The path of a traveler into the hole is shown by the curved line that passes through the event horizon. Two things stand out as unusual about this diagram: First there is an extra singularity in the past (at the bottom). Secondly, there is the extra universe on the left.

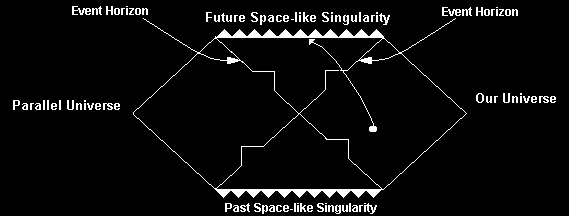
Figure 6: Penrose Diagram of Schwartzchild Black Hole (13)

The additional singularity, marked as past space-like singularity on the diagram, is what is known as a white hole. Very simply put, a white hole is the opposite of a black hole. Instead of engulfing everything that comes near it, the white hole repels matter. Notice the direction of its event horizon. Some physicists maintain that the singularity of a black hole opens into another universe (figure 7) . The idea behind a white hole is that matter that falls into a black hole in our universe is then belched out in another. It is worth noting, however, that astronomers have never observed a white hole, so their existence is doubted.

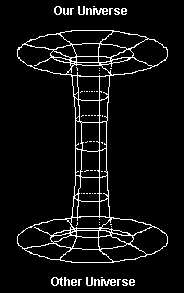
Figure 7: Black Hole Connected to Another Universe (14)

Figure 8 is a Penrose diagram of a Kerr black hole. It will be remembered that the spinning black hole exhibits several curious features, such as the ring singularity, the region of negative space, and the region through which travels into the past are possible. The ring singularity is denoted by the rounded off sharks teeth to show that it is a bit more forgiving than its non-rotating cousin. It should be obvious that the singularity is vertical (time-like). This means that one could escape from it with slower than light velocities. The area marked negative closed time-like loop is a region just inside the singularity. Very simply put, this is a region in which the normal barriers between past and future lose meaning. A traveler into this region could visit any place in his past or future, were it not for the one way nature of the event horizon. Just beyond the time-like loop region, lies the area of negative space. Unfortunately, this hole has not one, but two one way event horizons that would prevent a traveler from ever re-entering our own universe. However, as shown in the diagram, he would have his choice of many other universes to visit. Figure 8 shows four (three parallel universes, in addition to our own), however, this diagram could be extended an infinite number of times in both the past and future directions. Two examples of a travelers path into the Kerr hole are shown in the diagram. Path "A" takes the traveler into the ring singularity, while path "B" shows his path into another universe.

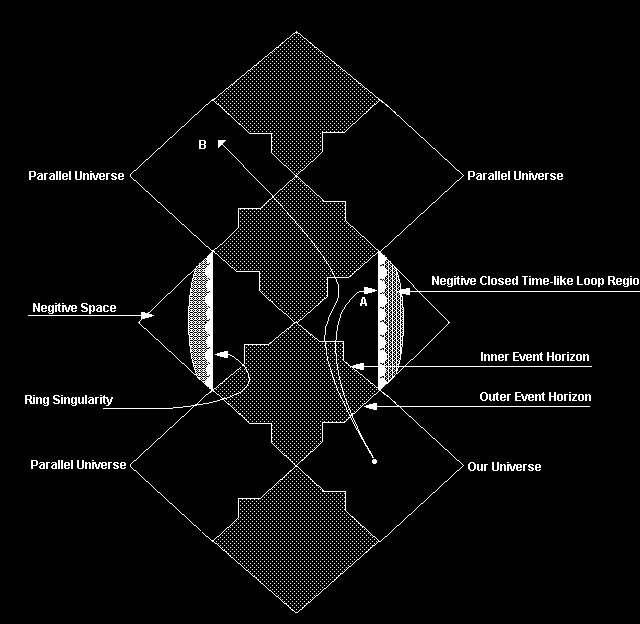
Figure 8: Penrose Diagram of a Kerr Black Hole

Do Black Holes Really Exist?

If a black hole, by its very nature, absorbs all matter and radiation that comes near it, then how would it ever be possible to detect one, short of falling into one? Astronomers have detected convincing evidence for the existence of several of these objects during the last 15 years thanks to the advent of x-ray telescopes. A black hole with another star in orbit near to it should be rapidly drawing matter from the star into it. The matter would circle the black hole in much the same manner as water going down a bathtub drain. This vortex of infalling matter is known to astronomers as an accretion disk. As the infalling stellar matter came closer to it's final destination at the event horizon, it would accelerate at ever increasing rates. The matter would begin to heat up, releasing photons of electromagnetic energy. Very close to the end of it's journey into oblivion, the stellar gasses would emit x-rays of intense energy. Astronomers search the sky for powerful x-ray sources that don't have a visual counter-part. Once an x-ray source has been located, the scientists try to determine if this source is a part of a binary star system (15). If the x-ray source is found to be part of a binary star system, astronomers can then calculate the mass of the unseen companion. This is done from spectral and visual analysis of the visual component of the binary system. Once it can be determined how long it takes the star to orbit the x-ray source, the mass of the source can be determined. If the x-ray source is found to contain, at a minimum, three times the mass of our sun, then astronomers are reasonably confident that a black hole has been discovered. To date, three objects that meet the above criteria have been discovered: Cygnus X-1, LMC X-3, and an x-ray emitter that has been recently discovered, known only by the designator A0620-00. Astronomers continue the search.

Conclusion

Black holes were first discovered, not in outer space, but in the brilliant solutions to Einstein's equations. To date, most of our knowledge of these mysterious objects remains theoretical. The distortions in the structure of space-time caused by these objects give rise to many strange and almost inconceivable phenomena. We have explored but a few of the more outstanding examples, such as the region of negative space within the ring singularity of the Kerr black hole, the inability of light to escape from within these objects, and the possibility of parallel universes. Astronomers and physicists believe that these objects are created as a result of the death of massive stars. This theory is beginning to be proven, as objects have been recently discovered that can only be explained using the theory of black holes. In addition to the highlights of this fascinating topic that we have explored, there are many other equally strange and wonderful possibilities lurking inside the shadowy world beyond the event horizon. Among them are the possibility of using a black hole as a source of energy that would dwarf the efficiency and energy output of even a thermonuclear reaction, microscopic black holes that actually lose mass, and super massive black holes that are thought to be at the centers of galaxies. The interested reader is invited to consult the materials listed in the bibliography.

(1) During the nineteenth century, it was shown by the Scottish physicist, James Clerk Maxwell that electromagnetic waves should exist, and that they would travel at approximately 300,000 km per second, the speed of light. Since all other waves required a medium in which to travel, it seemed inconceivable to Maxwell that electromagnetic radiation should be any different. Thus Maxwell reintroduced the idea of an ether, which served as the omnipresent carrier of these waves. The Michelson-Morely experiment was performed in an effort to detect this ether. The experiment failed repeatedly to find any evidence whatsoever that the ether existed.

(2) Einstein, Albert. Relativity: The Special and General Theory. New York, NY.: Crown Publishers, Inc., 1916. pp. 113-114

(3) Nicolson, Iain. Gravity, Black Holes, and the Universe. London: David & Charles, 1981. pp. 117 - 130.

(4) (ibid)

(5) (ibid, pp. 131 - 138)

(6) (ibid, p. 132)

(7) The terms time-like and space-like refer to travel that is slower than light or faster than light (forbidden), respectively.

(8) Herbert, Nick. Faster Than Light: Superluminal Loopholes in Physics. New York, NY: Penguin Books, Ltd., 1988. pp. 119 - 123.

(9) (ibid p. 125)

(10) Quantum mechanics is the branch of physics that deals with matter on a sub-atomic level. Its cornerstones are the Schrodinger Wave Equation, the Heisenberg Uncertainty Principle, and the Einstein-deBroglie Equations. Schrodinger's equation describes the nature of subatomic particles as waves. The Uncertainty Principle states that a particle's momentum and path can't simultaneously be observed. Einstein and deBroglie demonstrated that matter can exist as both a wave and a particle. These theories, and their successors, have combined to show us the quantum world. It is a strange world, ruled by probabilities. Very simply, from a quantum perspective, all possible outcomes of a situation exist side by side until the situation is observed.

(11) (ibid, Nicolson, p. 127)

(12) (ibid, Nicolson, p. 127)

(13) (ibid, Herbert, p. 122)

(14) Wolf, Fred Alan. Parallel Universes: The Search for Other Worlds. New York, NY.: Simon and Schuster, Inc., 1988. p. 160

(15) A binary star system is a system of two (or more) stars in orbit with each other. This is very common in the universe.

Bibliography

Herbert, Nick. Faster than Light: Superluminal Loopholes in Physics. New York, NY: Penguin Books, Ltd., 1988.

Einstein, Albert. The Meaning of Relativity. Princeton, NJ: Princeton University Press, 1953.

______. Relativity: The Special and General Theory. New York, NY.: Crown Publishers, Inc., 1916.

Naber, Gregory L. Spacetime and Singularities: An Introduction. New York, NY.: Cambridge University Press, 1988.

Nicolson, Iain. Gravity, Black Holes, and the Universe. London: David & Charles, 1981.

Schutz, Bernard. A First Course in General Relativity. New York, NY.: Cambridge University Press, 1986.

Wolf, Fred Alan. Parallel Universes: The Search for Other Worlds. New York, NY.: Simon and Schuster, Inc., 1988.